basic explanation of quantum physics
Now for a basic explanation of quantum physics and quantum mechanics. The first place to start would be to state what exactly quantum mechanics is. Simply put, quantum mechanics is the study of atoms and atomic structures. Note that these objects are VERY small. They are approximately the nano (10-9) to pico (10-12) scale.
Now to cover the basics of the subject. The first thing to discuss in a basic explanation of quantum physics is a mathematical construct called the wave function. In the simplest way possible, a wave function is a mathematical equation that represents all possible states subatomic particles (typically electrons) could be in. Here’s an example of a wave function.
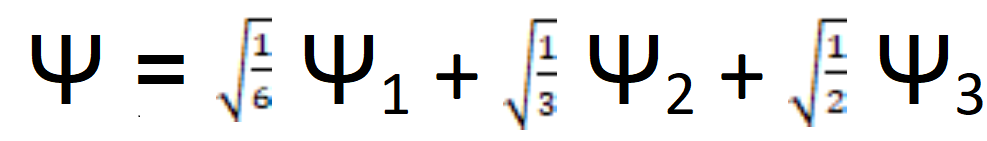
Do not feel intimidated by this!!! In the above figure, the wave function has been split up into three smaller wave functions (By the way smaller does not physically mean smaller. I’m using this terminology to help the layperson improve their understanding. Do not hate me for using such imprecise language.). Each of these smaller functions have their own distinct energy, momentum, etc. The square of the numbers in front of these wave functions gives you the probability the particles represented by the total wave function will be in the smaller states.
For instance, the square of the first wave function’s coefficient is 1/6. 1/3 and ½ are the square of the second and third functions respectively. When you add them together, 1/6 + 1/3 + ½ = 1= 100%. This makes sense. The particle(s) have to be in one of these states all the time.
Basic explanation of quantum physics: Quantization
In classical mechanics, the branch of physics that describes our everyday world, energy, momentum, position, and other similar quantities can have any value. For instance, when analyzing a falling object, we examine its energy. For argument's sake, at the top of it's motion, before it falls, it has a kinetic (or moving) energy of zero. Then, a millisecond before it hits the ground, it has a kinetic energy of 3 J (J=joules, a unit of measurement for energy). During the ball's motion, it takes all energy values between 0 J and 3 J. For instance, it can be 1.023487, 2. 23840, 2.999999999, etc. In other words, the energy has a continuous range between 0 and 3; it takes on all values between those numbers.
In contrast, quantum mechanics is discrete. Let’s say that the energies associated with the 3 smaller wave functions are 2.01 J, 7.723 J, and 8.1298 J. This means that the quantum particles represented by the big wave function can only have these energies. This quantization and discretization applies to position, momentum, etc. Click here for more information on the topic.